What Best Describes the Orthocenter of a Triangle

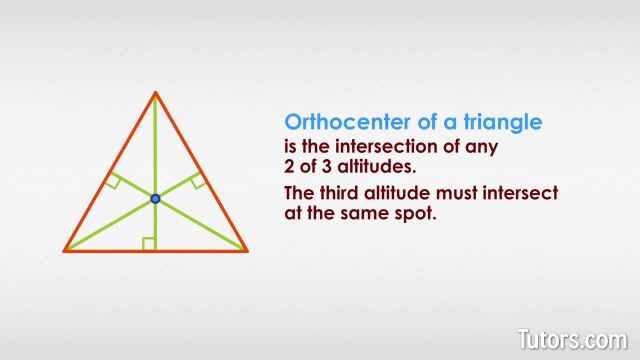
The orthocenter is one of the triangles points of concurrency formed by the intersection of the triangles 3 altitudes. The orthocenter of an acute triangle lies inside the triangle The orthocenter of an obtuse triangle lies outside the triangle The orthocenter of a right-angled triangle lies on the vertex of the right.

How To Find Orthocenter Of A Triangle 4 Easy Steps Video

For an acute angle triangle the orthocenter.

. These are supposed to be easy but i dont get it. The orthocenter is the point where the 3 altitudes of the triangle intersect. Find the equation of the 2 altitudes use the vertex and the slope of the altitude y y1 m x x1 6.

Liam calculated the weight of 3 different animals on his calculator and recorded the results below. It turns out that all three altitudes always intersect at the same point - the so-called orthocenter of the triangle. Set the 2 equations of the altitudes equal to each other and solve algebraically for x and y.

Orthocenter of a Triangle. The orthocenter of a triangle is the intersection of the triangles three altitudes. Find the equations of two line segments forming sides of the triangle.

The orthocenter of a triangle is the point where the perpendicular drawn from the vertices to the opposite sides of the triangle intersect each other. The orthocenter H of a triangle is the point of. It has several important properties and relations with other parts of the triangle including its circumcenter.

The orthocenter of a triangle is the point where the perpendicular drawn from the vertices to the opposite sides of the triangle intersect each other. The incenter of a triangle is the point at which the 3 medians lines from the vertex to the middle of the side opposite the vertex of the triangle intersect. Definition of the Orthocenter of a Triangle.

These three altitudes are. The orhocenter of a triangle is the point where the altitudes from the 3 vertices of the triangle meet. Equal distant from each side of the triangle NOT ON EULERS LINE Makes a circle in the triangle.

The orthocenter is the point of concurrency of the altitudes in a triangle. If VX9 find VY and YX. Let H be the orthocenter of OAB.

4 none of these. Find the slopes of the altitudes for those. The orthocenter is the point where all three altitudes of the triangle intersect.

A point of concurrency is the intersection of 3 or more lines rays segments or planes. The orthocenter is just one point. If the triangle is obtuse it will be.

A segment drawn through the midpoint of a side and at a right angle to the side. An altitude is the line drawn form one of the vertices of the triangle at right angles to the line. The orthocenter is not always inside the triangle.

The Orthocentre Of A Triangle With Vertices 0 0 3 4 And 4 0 Is. You can find where two altitudes of a triangle intersect using these four steps. The point when all three perpendicular bisectors meet.

An altitude is a line which passes through a vertex of the triangle and is perpendicular to the opposite side. Let O0 0 A 4 0 and B3 4 be the vertices of the triangle.

How To Find Orthocenter Of A Triangle 4 Easy Steps Video

How To Find Orthocenter Of A Triangle 4 Easy Steps Video

How To Find Orthocenter Of A Triangle 4 Easy Steps Video

How To Find Orthocenter Of A Triangle 4 Easy Steps Video